Icosahedron
Read here about the icosahedron, one of the Platonic polyhedra, and the dual of the dodecahedron. Some of the most significant tensegrity structures are argued to be congruent to the icosahedron.
Overview
The icosahedron polyhedron has 30 edges, 20 faces and 12 vertexes. A tensegrity that outlines this polyhedron has 6 struts and 24 tensile vectors.
This tensegrity structure is one of the few tensegrities that exhibit mirror symmetry. Burkhardt wrote, its "tendon network would mark out a cuboctahedron if the (non-planar) quadrilaterals in which the struts are nested were changed to squares. The struts are inserted as the diagonals of these squares so each strut is parallel to the strut in the opposite square and so no strut shares a vertex with another strut. This tensegrity is classified as a “diamond” type because each strut is surrounded by a diamond of four.
 Image width="273" height="220"
caption="Tensegrity structure of 6 struts and 24 tensile vectors that conforms to the classic icosahedron. From Practical Guide to Tensegrity Design 2nd edition © 2008 by Burkhardt "
Image width="273" height="220"
caption="Tensegrity structure of 6 struts and 24 tensile vectors that conforms to the classic icosahedron. From Practical Guide to Tensegrity Design 2nd edition © 2008 by Burkhardt "
The icosahedron-like tensegrity structure is argued to be the most significant of all the structures, particularly by researchers in bio-tensegrity of the fascia and muscle system. For example, see the right upper human extremity modelled as a sequence of interconnecting icosahedral tensegrities with compression struts of different lengths, below.
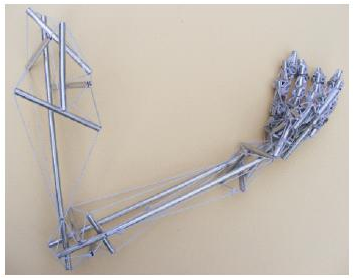 caption="Right upper extremity modelled as a sequence of interconnecting icosahedral tensegrities with compression struts of different lengths. Model: Graham Scarr as reproduced in Simple Geometry in Complex Organisms 2010. http://www.tensegrityinbiology.co.uk/publications/geometry/."
caption="Right upper extremity modelled as a sequence of interconnecting icosahedral tensegrities with compression struts of different lengths. Model: Graham Scarr as reproduced in Simple Geometry in Complex Organisms 2010. http://www.tensegrityinbiology.co.uk/publications/geometry/."
Comparing the icosahedron to other tensegrities
Motro published a detailed comparison between the polyhedron and its associated tensegrity structure. “The two geometries can be compared on basis of the ratio between the length of struts “s” and the distance between two parallel struts “d”. For the icosahedron this ratio is equal to approximately 1.618 (that is the “golden” ratio), for the associated tensegrity system it is equal to exactly 2. This resulting tensegrity system can be seen as the expansion of an octahedron, since there are at the end eight triangles of cables (the same as the number of triangular faces for an octahedron), and the three pairs of struts can be understood as the splitting of the three internal diagonals.”
 caption="Comparison between the icosahedron polyhedron and its associated tensegrity structure. “S” is phi, the golden ratio. From Structural Morphology Of Tensegrity Systems by Motro."
caption="Comparison between the icosahedron polyhedron and its associated tensegrity structure. “S” is phi, the golden ratio. From Structural Morphology Of Tensegrity Systems by Motro."
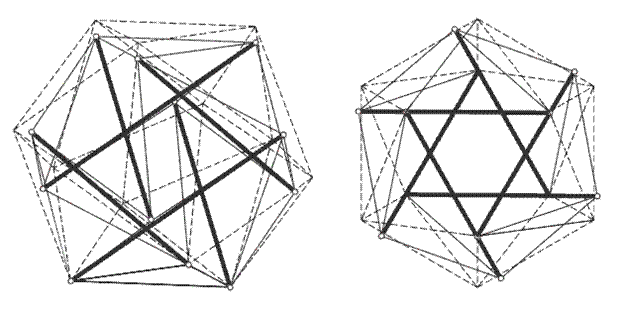 caption="Comparison of a tensegrity icosahedron and a polyhedron with which it conforms. Side view, left, and top view, right. The polyhedron is drawn with dashed lines. From Deployable Tensegrity Structures for Space Applications by Tibert. "
William Brooks Whittier also discusses this tensegrity, calling it a T-6. It has 6 struts and 24 tensile vectors. It outlines an icosahedron with 30 edges, 20 faces, and 12 vertexes.
caption="Comparison of a tensegrity icosahedron and a polyhedron with which it conforms. Side view, left, and top view, right. The polyhedron is drawn with dashed lines. From Deployable Tensegrity Structures for Space Applications by Tibert. "
William Brooks Whittier also discusses this tensegrity, calling it a T-6. It has 6 struts and 24 tensile vectors. It outlines an icosahedron with 30 edges, 20 faces, and 12 vertexes.
 caption="Tensegrity structure that outlines an icosahedron. In order from left to right two perspective views and a top view perpendicular to one strut pair. From Kinematic Analysis of Tensegrity Structures By William Brooks Whittier"
Gómez Jáuregui published a photo of a tensegritoy model he built that conforms with a truncated icosahedron.
caption="Tensegrity structure that outlines an icosahedron. In order from left to right two perspective views and a top view perpendicular to one strut pair. From Kinematic Analysis of Tensegrity Structures By William Brooks Whittier"
Gómez Jáuregui published a photo of a tensegritoy model he built that conforms with a truncated icosahedron.
File:Truncated icosahedron tensegritoy by Jáuregui.png
caption="Tensegritoy model that conforms with a truncated icosahedron. From Appendix G Tensegrity Models, Tensegrity Structures by Jáuregui"
Motro discusses a tensegrity structure related to the icosahedron. He wrote, “Since it is not possible to design a regular icosahedron with six equal struts, we tried to build one with six struts, one of them being greater than the five others. The basis of this design is a prismatic pentagonal system; a central strut is placed on the vertical symmetry axis. This axis becomes a rotation axis. The lengths of the struts and of the cables are calculated in order to reach an equilibrium state which is characterized by the fact that the twelve nodes occupy the geometrical position of the apices of an icosahedron. The name is chosen by reference to this axis of rotation and to the icosahedron. This system can be classified as a “Z” like tensegrity system according to the classification submitted by Anthony Pugh. There are only two cables and one strut at each node, except for the central strut.
 caption="Spinning icosahedron tensegrity structure, perspective and plane view. This can be classified a “Z” tensegrity system in the Pugh system. From Structural Morphology Of Tensegrity Systems by Motro"
caption="Spinning icosahedron tensegrity structure, perspective and plane view. This can be classified a “Z” tensegrity system in the Pugh system. From Structural Morphology Of Tensegrity Systems by Motro"
Discussion of the importance of the icosahedron
Is the icosahedron tensegrity the critical, primitive building block of biological tensegrity?
Pars wrote: The icosahedron-tensegrity has unique characteristics that tensegrities normally never have: The struts are exactly parallel or in straight angle to eachother. This is very rare for a tensegrity which can be distinguished by its twisted form. I think it may be these straight angles that make this tensegrity so popular. Wherever we humans changed the world we used straight angles, and although it may not be a natural form, for us a straight angle is restful and feels normal. I guess one could say that from all tensegrities the icosahedron tensegrity looks the most like a conventional construction. I made quite a few tensegrities, but the icosahedron was the first, also because mathematics behind it is more easy than for instance the 3 strut prism.
But all this doesn't say anything about nature or biotensegrity of course. If you ask me, I guess nature is full of tensegrities and the icosahedron is the bridge between nature and it's construction methods and us humans with our conventional bricks..
Levin: My understanding of Fuller: there are only three structures that are stable with flexible joints, the tetrahedron, octahedron and icosahedron. Therefore, biologic structures,( all joints from cell to organism are flexible, held by surface tension, integrins, mucus, ligaments etc.), must be constructed from some combination or permutation of those structures. For reasons previously stated, icosahedrons are the most biologically suited, closest packing, volume for surface area, least energy, etc. Icosahedrons are the most symmetrical of all polyhedrons, it is the most symmetrical system for the subdivision of a spherical surface into modular units and all spherical biologic structures must be icosahedrons to be stable or propped up by the adjacent structure so that, together, they create a stable structure,(fractals?), like bubbles in a foam.
The reasons, (amongst others), the icosahedron-tensegrity would work in biotensegrity is because it close packs and is self-organizing, and the most symmetrical, not because of the parallel struts. It is omnidirectional and has the largest volume for surface area and is the most energy efficient structure with the above characteristics. The icosahedron has already been recognized as the basic structure of carbon 60, viruses, cells, and much more in biology, (there are now well over 2000 scientific articles linking tensegrity and biology). 3-strut tensegrities have a larger surface area for volume, therefore, less energy efficient, and I don't think they close pack. I doubt if they would be self-organizing in nature.
Burkhardt: I can't see the tensegrity icosahedron as a building block for cell structure. But maybe this is just a question of semantics. Bucky did look at it as a building block, for example in 784 of Synergetics I], but I can't see that work as a model for a cell. For a model of cell walls I would be more comfortable with an approach that talks about a pattern of which the t-icosa (or perhaps della Sala's t-tetrahedron) is the simplest example. Bucky's development of that pattern into more complex single-layer domes or spheres is perhaps a suitable analogy for a cell wall. This approach is what I see in 717.01 of Synergetics I], but there is a variation here in that the tendons also appear to be attached to the middle of the strut.
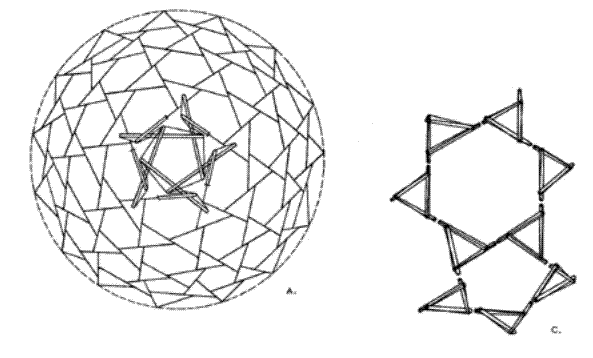 caption="Detail of Synergetics Fig. 717.01 Single and Double Bonding of Members in Tensegrity Spheres. Copyright © 1997 Estate of R. Buckminster Fuller "
caption="Detail of Synergetics Fig. 717.01 Single and Double Bonding of Members in Tensegrity Spheres. Copyright © 1997 Estate of R. Buckminster Fuller "
Certainly the pattern of the t-icosa has been developed more directly into higher-frequency structures without the tendons being attached to the middle of a strut. This is the approach Kenner develops, and also it is the approach of some of the tensegrity spheres developed by Bucky and his collaborators, although the latter that I see in Dymaxion World of Buckminster Fuller seem to favor the zig-zag approach of della Sala's t-tetrahedron, rather than the diamond approach represented by the t-icosa.
Unlike close packed spheres, close packing of icosahedra is not well defined
The notion of close-packing icosahedrons is problematic. de Jong notes: I don't feel that this has been described explicitly enough. Steve states that they close pack, like spheres where the middle one surrounded by twelve others nicely shrinks to accommodate, but the description of how one touches the next seems to be overlooked. Two tensegrities join to become a single tensegrity really only when cables meet bars and vice versa.
Real Icosahedron
The outline of the six-strut tensegrity as described above does not match the icosahedron as one of the platonic solids because the ratio between the length of struts “s” and the distance between two parallel struts “d” is exactly 2, where for the icosahedron this ratio is equal to approximately 1.618 (that is the “golden” ratio). There has thus been much controversy over the years about the use of this term in tensegrity, with many authors preferring to label it as an expanded octahedron.
Borge Jessen described a shape that he referred to as the Orthogonal Icosahedron, where all the faces meet at 90 degrees and match the outline of this 6-strut tensegrity. The card model shown expands and contracts within a similar range to the tensegrity icosahedron. Jessen’s icosahedron is described in:
Wikipedia YouTube Wolfram Maths although a correction to the latter is reported here with more detailed discussion here.
Not very well-known are the two tensegrities shown below and invented by Pars. The outside of these structures are exact icosahedrons.
File:Http://www.tensegriteit.nl/afbeelding/tensegrity210.jpg Image width="358" height="350" File:Http://www.tensegriteit.nl/afbeelding/tensegrity207.jpg The tensegrities can also be seen on Marcelo Pars' icosahedrons. 3D images of this real icosahedron are shown on Pars' 3D images] . Here a small picture of the icosahedron tensegrity without the struts:
File:Http://www.tensegriteit.nl/afbeelding/vmrlicosahedron03.png Image width="329" height="211"
